Mathematical Insights into Political Science (08/18/05)
Prepared by:
Joseph Malkevitch
Mathematics and Computing Department
York College (CUNY)
Jamaica, NY 11451
Email: malkevitch@york.cuny.edu (for additions, suggestions, and corrections)
Everyone knows that mathematics is a powerful tool for engineers, physicists, and chemists. However, it is less widely known that mathematics is valuable in all disciplines. This includes many subjects that some people think will not benefit from mathematical insights. Even a subject such as English has benefited from the use of mathematics. For example, recently mathematical tools were used to understand the origins of the Canterbury Tales. No original manuscript has come down to us, but using ideas about distance and phylogenetic trees, the relative "closeness" of the different manuscripts that have survived to the present can be studied.
What are some of the mathematical insights into political science? Among the social sciences other than economics, political science has perhaps benefited the most from the use of mathematics. Statistics is a branch of mathematics and when I use the term mathematical applications to political science here, I am specifically interested in non-statistical applications. There are many statistical applications as well.
Here are some examples:
1. Voting and Elections
Nearly all elections in the United States use plurality voting. Mathematical thinking shows that election systems can be modeled by selecting :
a. a ballot type, the instrument via which voters express their preferences among a collection of candidates or alternatives.
b. an election decision method which translates the voter preferences provided by the ballots into a winner, a collection of winners, or a ranking for the alternatives (which might be the candidates).
Mathematics has provided dramatic insights into this process. There are two stunning results:
a. Arrow's Theorem: For ranked ballots involving three or more candidates there is no election decision method which obeys all the fairness axioms (rules) in a reasonable list of such axioms.
Note: Arrow was an undergraduate mathematics major at City College before it became a part of CUNY. He continued his studies at Columbia University in Economics, and eventually won the Nobel Memorial Prize in Economics for his work on what is now known as Arrow's Theorem.
b. Satterthwaite-Gibbard Theorem: For ranked ballots with three or more candidates there is no decision method (other than dictatorship) which is immune from the value of strategic voting.; i.e. when voters have information about how other voters will be voting, they can improve their own chances in terms of the outcomes by misrepresenting their true preferences when they vote. Ideally, one would want to have a system where voters express their preferences in an honest way.
Some have interpreted these theorems to mean that since one can not have a "perfect election system" plurality is as good as anything else. However, this is not the case. There are many ways to improve on plurality voting.
To help get you thinking about elections, who should win this election? When many candidates run, as is the case in primary elections, say, for the position of Democratic Party candidate for Mayor, there are often as many as 5 serious candidates.
Fifty-five voters (or scale this up by 100,000 if you want) have produced the following collection of ballots on 5 choices. The choices are named A, B, C, D, and E. The higher up letters are ranked more highly.
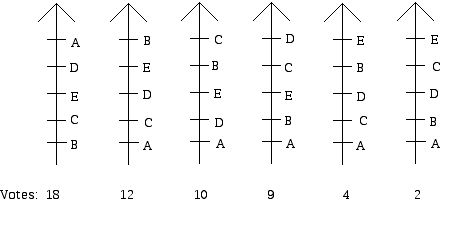
Other insights that mathematics has given into voting is via the use of power indices for weighted voting situations. The most famous weighted voting system for Americans is the Electoral College. Large population states carry larger blocks of votes in the Electoral College than smaller states. However, how much power does this give them? Weighted voting is also used extensively in the European Union. Not surprisingly, large countries such as Germany, France, Italy and England cast more votes (weight) than countries such as Belgium or Sweden. Two well known indices for measuring how much power a player in a weighted voting game has are those of John Banzhaf and Lloyd Shapley.
2. Apportionment
The apportionment problem aims to distribute a collection of objects, for example, the number of seats in the US House of Representatives, to a collection of claimants (e.g. the states) fairly based on their populations. In Europe the apportionment takes the form of giving each party a fair number of seats in parliament based on the number of votes each party gets. Many other problems fall under this category. The critical thing in the apportionment problem is that the items to be "shared" can not be divided into small parts such as water or food. The solution must involve whole objects.
Again mathematics has clarified the situation via the Balinski-Young Theorem. This theorem shows that an ideal apportionment system is impossible. There is a trade-off between giving the claimants their "quota" and monotonicity in the house size or the size of a claimant state's population.
Mathematics is also of use in understand the practice of gerrymanding, drawing district lines for a political advantage. The phenomenon of gerrymanding has grown in recent years.
3. Conflict Resolution (Game Theory)
In many situations there are "players" in conflict with each other who can take different actions. Depending on the joint actions of the players there is a payoff to the players. What is the wisest action to take? The theory of games, which was brought to prominence by a book written jointly by the mathematician John Von Neumann and the economist Oskar Morgenstern, who laid down the foundations of a mathematical theory for games. One big distinction between games is in which the "players" are able to cooperate and those in which they are noncooperative. Game theory ideas have given political scientists a powerful tool to examine a very broad collection of phenomena. For example, during the Cold War, study of the "paradoxical games" Chicken and Prisoner's Dilemma were very fruitful in trying to interact with dictatorships.
4. Insights into Warfare
It might seem as if equations could not be of use in studying warfare but Fredrick Lancaster, a British mathematician, used differential equations to get significant insights.
5. Fair Division and Fairness
Many problems in political science can be posed as fair division or fairness questions. How should a society tax its citizens fairly? How should the tax money that is collected by a government be used fairly for the benefit of its citizens? What principles of "distributive justice" should be used? Mathematical tools have been developed to measure the level of inequality within a country and attempts can be made to show how this measure is changing with time. For example, in the United States the rich are getting richer relative to other parts of the population at an increasing pace.
References:
Arrow, K., Social Choice and Individual Values, 2nd ed., Wiley, New York, 1963.
Balinski, M. and H. Young, Fair Representation, Second Edition, Brookings Institution, Washington, 2001.
Brams, S., Game Theory and Politics, New York, Free Press, 1975.
Brams, S., Rational Politics: Decisions, Games and Strategy, Washington, CQ Press, 1985.
COMAP, For All Practical Purposes, (6th edition, as well as earlier ones), W. H. Freeman, New York
Gibbard, A., Manipulation of voting schemes: a general result, Econometrica 41 (1973) 587-601.
Hodge, J. and R. Klima, The Mathematics of Voting and Elections, American Mathematical Society, Providence, 2005.
Luce, R. and H. Raiffa, Games and Decisions, Wiley, New York, 1957.
Malkevitch, J. , Mathematical theory of elections, in Mathematical Vistas, J. Malkevitch and D. McCarthy (eds.), Annal 607, New York Academy of Sciences, 1990, pp. 89-97.
McClean, I. and A. Urken, Classics of Social Choice, U. Michigan Press, Ann Arbor, 1995.
Moulin, H., Fair Division and Collective Welfare, MIT Press, Cambridge, 2003.
Saari, D., Decisions and Elections, Cambridge U. Press, New York, 2001.
Saari, D., Chaotic Elections, American Mathematical Society, Providence, 2001.
Satterthwaite, M., Strategyproofness and Arrow's conditions, J. of Economic Theory 10 (1975) 187-217.
Straffin, P., Topics in the Theory of Voting, Birkhauser, Boston, 1980.
Straffin, P., Game Theory and Strategy, Mathematical Association of America, Washington, 2001.
Taylor, A., Mathematics and Politics, Springer-Verlag, New York, 1995.
Taylor, A. and W. Zwicker, Simple Games, Princeton U. Press, Princeton, 1999.
Young, H., Equity in Theory and Practice, Princeton U. Press, Princeton, 1994.
Young, H., (ed.), Negotiation Analysis, U. Michigan Press, Ann Arbor, 1991.
Back to list of tidbits

